Book
Problem Solutions
Chapter
3 problem solutions
3.4:
Simplex
channel = 25kHz => Duplex channel = 50kHz
So
20MHz/50kHz = 400 channels.
If
N=4, using omnidirectional antennas => 400/4 = 100 channels per cell site
3.5:
We
must compute Qn/i0 for each case with Q=(3N)1/2
and n=4. This simplifies to (3N)2/i0. The 15 db criteria
means we need (3N)2/i0 > 31.62
i0
=6, so N>4.5 => N=7
a) i0
=2, so N> 2.65 => N=3 (theoretically… )
b) i0
=1, so N> 1.87 => N=3 (theoretically… )
So you
get a better N with sectoring. Also, there is no improvement in N going from
120 to 60 degrees so you will loose trunking efficiency with nothing to show
for it. The best choice is to use 120 degree sectoring (i.e. three sectored
sites).
(NB:
This is true regardless of whether you use the theoretical N=3 value or opt for
a more practical N=4 value).
3.8:
As
above with n=3 we need (3N)1.5/i0
> 31.62:
a) i0
=6, so N>11.006 => N=12
b) i0
=2, so N> 5.29 => N=7
c) i0 =1, so N> 3.33 => N=4
Now it
makes more sense to use 60 degree sectoring (i.e. six sector cells) since we
get a better value for N.
3.10:
Duplex
channel = 60kHz so 24MHz/60kHz = 400 channels. Au=0.1 E.
a) 400/4=100
per cell. Assuming old style AMPS/DAMPS we need 1 cntl channel per cell so that
leave 99 tch per cell.
b) Perfect
scheduling would be 99 circuits in use on each cell. 90% of that means 89.1
circuits in use so we have 99 channels serving 89.1E of users. Now if Au=.1E
this means we can serve 891 users per cell
c) From
the chart I gave in class 99 channels gives ~89.1E with GOS =0.03. or 3%
blocking probability (roughly)
d) With
120 degree sectoring, we get 33 channels per cell/sector on two sectors and 34
on the third for each basestation. So we
have 32 TCH and one CNTL per sector for two sectors and 33 TCH one CNTL for the
third on each basestation. So from the Erlang B chart: for 32 TCH channels at a
0.03 GOS we get 24.9E or 249 users and for 33 channels at 0.03 GOS we get 25.8E
or 258 users. This give a total of 756 users that each basestation can support.
e) 50x50 =
2500square km so if each basestation covers 5square km, this give 500
basestations. So in the omnidirectional case we can serve 500*891 = 445500
customers
f) 500*756
= 378000 customers.
3.11:
I am
interpreting 57 channels as meaning 57 channels per cell/basestation in the
omnidirectional case (If all you have is 57 channels for a system, I’d quit now
and take up knitting) I will also assume that (like AMPS) control channels are
in a separate part of the spectrum and the 57 refers to traffic channels only.
GOS=0.01 so in the omnidirectional case each cell can handle 44.22E. H=2min and
λ =1/hr so Au= 1/30 = 0.0333 E. Thus each cell can handle 44.22/.0333 =
1326.6 users (on average). With 60 degree sectoring we get 3 sectors with 10
channels and 3 with 9.
10
channels => 4.46E => 133.8 users while 9channels => 3.78E =>113.4
So the total users declines to 741.6 with sectoring.
3.13:
300
traffic channels (we assume control channels are handled separately) with N=4
=> 75 channels cell. 0.01 GOS =>
60.73E per cell. Au=0.04E => 1518.25 users per cell. For 84 cells
=>127533 total users on the system
N=7
=> 43 channels per cell for 6 cells
(one has 42). 0.01GOS => 31.66 (30.77
for one). Au=0.04 => 791.5 (769.25) =>
66219 users on the system
N=12
=> 25 channels per cell. 0.01 GOS => 16.125 E per cell. Au=0.04 =>
403.125 users per cell => 33862.5 users on the system.
3.15 is
not a good problem.
3.16:
We use
P/P0 = (d/d0)-n where P0 = 1 mW, d0
= 1m, n=3, and P=-100dBm = 10-10mw. So we get 1010 = d3
=> d= 103.333 = 2154.4 m =2.1544km. Now the ratio between the
distance between cells and the major cell radius D/R = Q=(3N)1/2.
So: R = 2.1544 / (3N)1/2 km so
N=7
=> R = ~470 m
N=4
=> R = ~622m
Chapter
4 problem solutions
4.1
Linear
version:
Pr = Pt
( λ/(4πd) )2 GtGr. λ=(300m/µs)/900MHz=0.333m d=1000m.
Gt=Gr=1
So: Pr
= 10 (0.333/4000π)2 =7.022x10-9 W
Decibel
version:
Pr = Pt
+Gt +Gr –FSL. Pt=40 dBm, FSL=20log(900) + 20log(1) + 32.45 =91.53
So Pr =
40 – 91.53 = -51.53 = 7.023x10-6mw=7.023x10-9W
4.3
The
gain of an antenna is given by G = Aη/(4πλ2) where
Aη is the effective aperture (as I mentioned briefly when deriving the FSL
equations). A is the area and η is the efficiency (usually between .4 and
.8) For the sake of this problem we can guess that η= ~0.5, which gives G
= ~26 dB. Forget the HPBW.
f=60GHz
so λ=(0.3m/ns)/60GHz = 0.005m.
The
largest dimension of the antenna is 0.046m.
So 2D2/λ
= 2(0.046)2/(0.005) = 0.846m =84.6 cm
4.4
1W = 30
dBm. Given the gain is 26 db (above), EIRP = 56dBm.
FSL = 20log(dkm) +20log(60000) +
32.45. RSL = EIRP – FSL + Gr =82 - FSL
So:
d=1m=
0.001km: FSL= 20log(0.001)+20log(60000) +32.45 = 68.01 RSL= 82 - 68=14dBm
d=100m=0.1km:
FSL = 20log(0.1)+20log(60000) +32.45 = 108.01 RSL = 82 – 108= -26 dBm
d=1000m=1km:
FSL = 20log(1)+20log(60000) +32.45 = 128.01 RSL = 82 – 128= -46 dBm
4.14:
50W =47
dBm. Gt=0dB, Gr= 3dB. f=1900MHz. d=10km.
FSL =
20log(10)+20log(1900)+32.45=118.03dB.
a) Pr= Pt
+ Gt –FSL + Gr = 47 +0 -118 +3 = -68dBm
d) FEL =
40log(10000) – 20log(50) – 20log(1.5) = 130.46 so
Pr= Pt + Gt – FEL +Gr = 47 – 130.46 +3 = -
80.46 dBm
4.19:
Pt=10W=40dBm.
Gt=10dB, Gr=3dB, L=1dB. So EIRP = 49dB and RSL = EIRP – PL + Gr
(normally
I take Gr to be zero for the usual cellular situation. However in this case,
they are assuming a car with a whip antenna, which will give an extra 3dB
gain…).
f=900MHz
so λ=.3333
We will
do this backwards: Total distance= 5 km. So:
FSL =
20log(900)+ 20log(5)+32.45=105.51 dB
So, RSL
without diffraction = 49 – 105.51 + 3 = - 53.51dBm
For
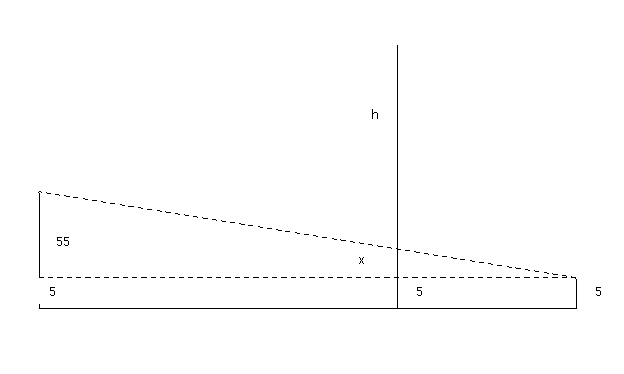
diffraction, we do the usual geometry stunt and get
400 = 5
+ x + h
where 5
is the height of the mobile, h is the height of the obstruction above line of
sight, and
x is
given by 55/5 = x/2. So x = 22m and h
=373m See the drawing below
Now
ν = h [ 2(5000)/(λ(2000)(3000)) ]1/2 = 373 ( 1/200)1/2
= 26.375
Using
4.61e we get
Gd =
20log(0.225/ν)= -41.38 dB
So RSL
with diffraction = -53.51 – 41.38 = -94.89 dBm
4.20:
We use the same equations:
a) f=50MHz => λ=6m FSL = 20log(50)+
20log(5) +32.45 = 80.41dB
RSL without diffraction = 49 – 80.41 +3 = -28.41 dBm
ν= 373[ 2(5000)/( 6(2000)(3000)) ]1/2=
6.2167
Gd=20log(0.225/ν)= -28.83 dB
RSL with diffraction = -28.41 -28.83 = -57.24 dBm
b) f=1900MHz
=> λ=0.15789m FSL=20log(1900)+20log(5)+32.45=112 dB
RSL without diffraction = 49 – 112 +3 = -60
dBm
ν= 373[ 2(5000)/( (0.15789)(2000)(3000))
]1/2= 38.3221
Gd=20log(0.225/ν)= -44.63 dB
RSL with diffraction = -60 – 44.63 =
-104.63 dBm
4.23:
This is
a challenging (and confusing) exercise in using P/P0 = (d/d0)-n
where P0= 1µW, d0 = 1km and d=2, 5, 10, and 20 km. The
value of n varies with the model:
a) Free
space => n=2
b) n=3
c) n=4
d) for the
approximate equation for FEL, n=4 as well
e) n will
be the coefficient of the log(d) term (divided by 10) =
[44.9- 6.55log(h)]/10 = 4.49 -.655log(40) =
3.44
|
P for
d= |
2km |
5km |
10km |
20km |
|
a) n=2 |
0.25
µW |
0.04
µW |
10nW |
2.5nW |
|
b) n=3 |
0.125
µW |
8nW |
1nW |
0.125nW |
|
c) n=4 |
62.5nW |
1.6nW |
0.1nW |
6.25pW |
|
d) FEL |
62.5nW |
1.6nW |
0.1nW |
6.25pW |
|
e) N=3.44 |
92.1nW |
3.94nW |
0.363nW |
33.45pW |
Chapter
5 problem solutions
5.1:
The
Doppler shift is given by fd = (v/λ)cos(θ). The maximum will be when
cos = +1 and the minimum will be at cos = -1 so we need only compute fm = v/
λ and then add and subtract. We must be careful of the units, however:
1km/hr = 0.2778 m/s . Also: λ =
3x108/1.95x109 =0.1538m and watch the significant digits:
a)
1km/hr
= 0.2778 m/s => fm = 1.8056 Hz => maxf = 1950.000002MHz minf = 1949.999998MHz
b)
5km/hr
= 1.3889 m/s => fm = 9.0278 Hz => maxf = 1950.000009MHz minf = 1949.999991MHz
c)
100km/hr
= 27.78 m/s => fm = 180.56 Hz => maxf = 1950.000181MHz minf = 1949.999819MHz
d)
1000km/hr
= 277.8 m/s => fm = 1805.6 Hz => maxf = 1950.001806MHz minf = 1949.998179MHz
5.8:
For
P5.6a:
We
have 4 components so
τ = [0(1) + 50(1) +75(.1) +100(.01) ]/[1+1+.1+.01]=58.5/2.11 = 27.725 ns
τ2
=[0(1)
+ 502(1) + 752(.1) + 1002(.01) ]/2.11 =
3162.5/2.11=1498.915 ns2
σ= (( τ2) - ( τ )2)1/2 = (1498.915 – 768.682)1/2= 27.02 ns
So
for 90% Bc = 1/(50*27.02) = 7.4x10-4
GHz = 740KHz (NB: t in ns => f in GHz)
For
50% Bc = 1/(5*27.02) = 7.4x10-3
GHz = 7.4 MHz
For
P5.6b:
We
have 3 components:
τ = [0(.01) + 5(.1) +10(1) ]/[1 +.1+.01]=10.5/1.11 = 9.459 µs
τ2
=[0(.01)
+ 52(.1) + 102(1)]/1.11 = 102.5/1.11=92.3423 µs2
σ= (( τ2) - ( τ )2)1/2 = (92.3423 – 89.4814)1/2 = 1.691 µs
So
for 90% Bc = 1/(50*1.691) = .0118 MHz =
11.8KHz
For
50% Bc = 1/(5*1.691) = .118 MHz = 118KHz
5.9:
Binary
modulated 25kbps signal => bit duration T = 1/25000 = 40 µs
To
run without an equalizer, we need to be in a flat fading environment, which
means we need
σ << T = 40 µs The book uses a rule of thumb which says
“<<” is about a factor of 10 so they get a max σ of about 4 µs
For
8PSK modulated at 75kbps we get 3 bits in every symbol. Thus the symbol rate is
25ksps so T is again 40 µs so the answer is the same as above.
5.13:
f
= 900MHz so λ=1/3 =0.333 m. We don’t know v, but we can find it if we get
the Doppler frequency from the afd. The afd = 1ms for a signal level (by which
I assume they mean power level) of 10 dB
below the rms level. So ρ = -5dB =
.316
afd
=( eρ2- 1)/[ρfm(2π)1/2] = 1ms =
0.001 s
So
fm = (e0.1 -1 )/[ ρ(2π)1/2(0.001)] =
132.68 Hz
So
v = fm/λ = 44.23 m/s
So
in 10s the vehicle travels 442.3 m
To
find the number of fades we need the level crossing rate: N= ρfm(2π)1/2
e-ρ2=95.16 or about 95
5.28:
a) τ
= [0(1) + 1(.1)
+2(1) ]/[1 +.1+1]=2.1/2.1 = 1 µs
τ2
=[0(1) + 12(.1) + 22(1)]/2.1 =
4.1/2.1=1.9524 µs2
σ= ((
τ2)
- (
τ
)2)1/2
= (1.9524 - 1)1/2= 0.9759 µs
b) all
components are > 20db below so after 2 µs we get everything so τmax
20dB= 2 µs
c) So we require
T > 10σ = 9.759 µs
=> Symbol rate <
1/(10σ) = 102.47 ksps
d) We must compute the coherence time so we need
the maximum Doppler shift.
v = 30km/hr = 8.333 m/s, f = 900 MHz
=> λ=c/f = 1/3 m so fm=v/
λ=8.333/.3333= 25Hz. Using the conservative value of Tc we get 7.16 ms.
Using the practical value we get 16.92ms. So depending on what “ highly correlated” means, you should get
one of these values
5.29:
f
= 6GHz => λ = 0.05 m. Since v = 80kph = 22.222 m/s, fm=v/λ=
444.44 Hz
a)
Zero
crossings about the rms value => ρ =1
so the lcr = N = ρfm(2π)1/2 e-ρ2
= 409.839 per second
So over 5 seconds you get 2049.19
b)
Afd =( eρ2- 1)/[ρfm(2π)1/2]
= 1.54 ms (NB: so on average the signal
spends about 63.2% of its time below the rms level. This is consistent with the
Rayleigh fading model)
c)
Again,
I assume 20 dB is a power level estimation so ρ = 0.1
then afd
=( eρ2- 1)/[ρfm(2π)1/2]
=90.2 µs
5.30:
We
look at each scenario:
a)
Urban
environment => slow mobiles, e.g.
v<30mph < 50kph < 14 m/s so fm < 46.33 < 50Hz
so even the conservative value of Tc is 3.58 ms. Data rate = 500kbps => Ts = 2 µs so the
fading is definitely not fast. However,
to pass a 500kbps signal, the channel would need a coherence bandwidth (using
the 50% coherence) of 500kHz so σ< 0.4 µs. This is not likely in an
urban fading environment (see , e.g. problem 5.28 or 5.8b above ) Thus this should be frequency
selective fading scenario.
b)
For
a highway environment v is much larger, so say v = 60mph so f may be 100Hz.
Using the practical value give Tc = 4.23 ms (conservative gives 1.8ms). Data rate of 5kbps => Ts = 0.2 ms so the
environment is still pretty slow (i.e. not a fast fading environment). However a 5 kHz signal requires only that
σ< 40 µs. This is a pretty easy requirement to meet for most channels.
Thus this is likely to be a flat fading scenario.
c)
At
10bps, Ts = 100ms. Tc is going to be much less than this (as describe in b and
a above) so the environment is clearly fast fading. ( the requirement on σ
will be on the order of milliseconds so the environment will also be flat)
Revised 3/12/12